Math tidbits and sometimes a little more.
Fun facts to know and tell.
Math tidbits and sometimes a little more.
Fun facts to know and tell.
Tuesday, April 30, 2013
Dr. Kenneth Appel and the Four Color Map Theorem: Part 2
When mathematicians look at the four color map problem, they often turn the regions into vertices or nodes - here represented by ovals - and draw a line between any two nodes whose regions share a border. This object is called a graph, and because none of the lines connecting nodes crosses any other, the specific type of graph is called planar.
Dr. Kenneth Appel proved that all such planar graphs can be painted with four or less colors such that no two nodes that have a line connecting them directly are the same color. If you think about any graph, you could always add a new node an a few lines from that node to some of the other nodes on the existing graph. The implication of this is that there are infinitely many graphs.
Here's the tricky part of Dr. Appel's proof and I will not try to present an explanation for it. There are ways to make one graph equivalent to another, which means that if one graph can be four-colored, the equivalent graph can be four-colored as well. Even though there are infinitely many graphs, Dr. Appel's method proved there are exactly 1,936 different equivalence classes.
It's not uncommon for mathematical proofs to have to several cases that have to be taken separately, but 1,936 is a lot of cases. Instead of doing each one by hand, Appel decided to write a computer program that looked at every case. When it was finished and it had looked at all the data, it had proved each of the cases could be color with no more than four colors and the proof was complete.
There were many mathematicians who were not satisfied with the new method, but there were many who saw this as a way to use computers to forward the field of mathematical inquiry. While the controversy is not yet over, Appel's proof is accepted and the use of computers in math research is much more common now than it was in the 1970s.
Best wishes to the family and friends of Dr. Kenneth Appel, from a fan.
Monday, April 29, 2013
Dr. Kenneth Appel and the Four Color Map Theorem: Part 1
Dr. Kenneth I. Appel died earlier this month at the age of 80. He is best known in mathematics four proving the Four Color Theorem, which states that any map of regions drawn on a flat piece of paper can be colored with no more than four colors in such a way that no two neighboring regions are the same color.
The proof was controversial in its day - the 1970s - because it used a computer to complete a vital but time consuming step.
Here is a map of the Western United States. Let me show a way to color it using four colors. It is not unique and the method I use causes a problem at the end, but by switching things around that problem can be solved.
An important idea in map coloring is that two regions are adjacent if they have a common edge, not if they meet at a common corner. In this example we have the four states that meet at a corner, In the same way a checkerboard can use two colors without people being confused, we can have Arizona and Colorado share a color, here signified by the number 1, and New Mexico and Utah share a different color, signified by the number 2.
Having done this, we now have states that border a 1 state and a 2 state, so I give all these states - Nevada, Wyoming and Oklahoma - the number 3.
Having done things in this method, I will now be forced to use a fourth color when mapping Kansas and Nebraska, and likewise there will be a forcing issue once, California, Oregon and Idaho are colored in. I also colored in Texas with a 1 since it was bordered by a 2 and 3.
I can easily finished the rest of the map, but this pattern has a problem. Imagine that some single region surrounds all these states. Because states on the outside edges have all four colors, this huge imagined state would have to be a fifth color, which would say four colors do not suffice. If I had started the problem knowing about that constraint, I could have still solved the new problem with four colors. The easiest way to do this changing as few states as possible is to switch the 3 and 4 in Wyoming and Nebraska, switch the 3 and 4 in Nevada and Oregon and change Washington to a 2. Then all the states on the outside have the numbers (colors) 1, 2 and 3 and the surrounding region can be given the color 4.
Tomorrow, I'll give a sketch of the proof, glossing over some difficult math and discussing the controversy of using computers in proofs.
I present this picture as tribute of the moment. Appel was at the University of Illinois when he published and the local post office commemorated his achievement with this postmark stating FOUR COLORS SUFFICE. It became a collector's item for mathematicians, like this postcard sent from the Urbana post office to Ulm, Germany while the postmark was being used.
Sunday, April 28, 2013
The Statistical World: Part 3, life and death
When discussing probabilities, the two common measurement systems are ratios and percents. A ratio would be written as 1 chance in 3 or a probability of 1/3. The same number as a percent would round to 33% or possibly 33.3%, which as a fraction would be 33/100 or 33.3/100. Going past a tenth of a percent in rounding is rare.
When discussing death statistics, the usual time period is a year and the usual scale is per 100,000 population. The most likely causes of death for all adults are heart disease and cancer, both currently slightly under 200 deaths be 100,000 population in the United States, according to The New England Journal of Medicine.
Heart disease is dropping quickly as a cause of death. In 1960, it killed 369 of every 100,000 people, nearly twice the rate we see today. Cancer, on the other hand, is rising slowly. It went from 149 of 100,000 in 1960 to 186 of 100,000 in 2010. The general consensus is that we are seeing more cancer deaths because people are living longer and not dying of other diseases.
And then there is death by violence. In the general population, it is a much smaller risk than the major diseases. In 2010, accidents caused 38.2 deaths per 100,000 population, making it the fifth most common killer. The only other death by violence in the top ten these days is suicide at 12.2 per 100,000. The murder rate is much lower.
I show a picture of a gun here because of the noticeable dichotomy in suicide rates between men and women. Women's suicide rate is less than 5 per 100,000 while men's rates about 19 per 100,000. That would seem to say men are about four times more likely to kill themselves than women are, but that is not the entire picture. When looking at reported suicide attempts, women try to kill themselves at a rate three times greater than men's. Multiplying three by four, this means a man attempting suicide is twelve times more likely to succeed than a woman.
Men attempting suicide are much more likely to use a gun than women are. For all the fear we have of mass killers, the biggest public safety problem concerning guns happens alone behind closed doors.
I apologize here midway through this post for morbidness, but it is about to get worse. Now we look at infant mortality, babies born alive but not surviving to a first birthday. Instead of being counting on the scale of 100,000, infant mortality is counted per 1,000 live births.
In 1960, infant mortality was much worse than it is today, even in industrialized nations. The United States was losing 26 of every 1,000 babies, which would round 2.6% By reports I have seen, it was significantly worse in 1956, the year infant mortality mattered to me personally, though I was blissfully unaware.
Unaware, but not untouched. When I was a baby, I contracted bronchialitis, an inflammation of the small blood vessels in the lung. There have been attempts to find a cure, but now as then, for the most part this is a watch and wait situation. I was fed through a tube in my heel and - spoiler alert - I survived. The scar, once very noticeable, is now high up on my ankle.
This graph shows six countries arbitrarily chosen from among the industrialized world: France, Ireland, Sweden, Switzerland, the United Kingdom and the United States. Even in 1960, we were not "number one", as we so often have been taught to think of ourselves. Now we are in sixth place out of these six.
This is not random chance. Every country we think of as being "advanced" or "industrialized" does a better job of keeping babies alive. The countries we compete with are like Croatia and other Eastern European still trying to catch up after decades of living under backward Communist rule. (Notice that the countries ahead of us all practice what is called socialism by American standards.)
Infant mortality is a difficult and complex subject. It is getting better, even in the worst places, but even in the best places that are around 2 per 1,000 live births lost, that would multiply out to 200 per 100,000. it's as bad as heart disease is for the general population.
Infant mortality is not part of the general conversation in this country. It should be and it should not be a cause of conflict culture, one political side against another. We have the means to do better. We only need to add the political will.
Saturday, April 27, 2013
The Statistical World: Part 2
As someone who use to gamble much more than I do now, I have a visceral understanding of probability that non-gamblers do not have.
For example, if we reach this position in a game of backgammon, here are the odds of winning.
If it's black's turn: game over. Black wins 100% of the time.
If it's White's turn: There are exactly 19 rolls that win and 17 rolls that lose. It's a little better than flipping a fair coin, which would be 50%-50%. This rounds to 52.8% chance to win and a 47.2% chance to lose.
The only thing to question is if "fair dice" exist, or even "fair coins". Lots of data has been compiled and the answer is yes. Most coins in your pocket will come up heads when flipped about 50% of the time, and most dice have a roughly even distribution of the six possible numbers.
And then there is randomness in the real world. if you saw the first episode of Mad Men, you might recall that the Surgeon General's 1960 report on the effects of smoking and the subsequent publishing in Reader's Digest was a major plot point. To drive home the point that Don Draper is not just a pretty face, he comes up with the Lucky Strike slogan "It's toasted" on the spot when pitching to the worried owners.
If fact, the slogan is real and pre-dates the 1960 advertising crisis by several years. Mad Men's first episode gets several things right, including that the tobacco industry would be barred from using testimonials from doctors or even dentists in the years to come.
But what are the exact probabilities of cigarette smoking causing you harm and shortening your life? Here the numbers get hazy. Many different factors can be considered other than smoking, some that make things better and some that make things worse. It is about as far as you can get from an exact science, but serious experts take randomness into account and still claim smoking has multiple ways it can screw up your health, many of them very significant changes for the worse indeed.
Tomorrow, we talk about the randomness.
Friday, April 26, 2013
The statistical world: Part 1
We live in a statistical world. What I mean is that for most of the decisions we face, there are some choices that are better than others, but they do not guarantee success or even that minimal level of success, breaking even.
Tic-tac-toe is part of the mathematical world. In the mathematical world we have proof, a guarantee of success or a guarantee of failure. If two player are at the maximum skill level in tic-tac-toe, every game is a draw, because the first player puts his or her mark in the center and the second player puts the opposite mark in one of the four corners.
Not every game in the mathematical world ends in a draw. In the simple number game "How much money does your dad make?", the first player names a number and the second player can always name a higher number. There are other simple games where going second is a forced win, like certain variations of Nim.
Yahtzee is the commercial name for a game that has been around for a long time. It is more involved than tic-tac-toe, but at the end of the 20th Century computer scientists "solved" the game, meaning they found the optimal play in every possible situation, meaning every possible roll with even possible combination of scores you have already achieved. If you were to match two computers perfectly programmed, you would not expect a draw in every game. In fact, actual tie scores should be extremely rare. What you would expect in the very long run is that both sides would win equal numbers of games, regardless of who played first or second. (Yahtzee is really a solitaire game, though usually played in groups. My dice rolls do not effect yours and vice versa, though some players might make decisions because they are ahead or behind late in a particular game that they would not make in a true solitaire game.
Even though we know the best way to play, it does not insure victory or even a draw every time. Randomness is so great in Yahtzee that the best possible strategy might lose to some other strategy if both players are promised to roll the same values in all cases until the game ends.
This is what I mean by the statistical world. Risks and rewards may or may not be completely understood, but even if we know the odds down to exact values, randomness can produce very strange results. Tomorrow, I will discuss the idea of assessing risk, something we do not always do very well.
Wednesday, April 24, 2013
U.S. vs. the Rest of the World:
Climate change as measured from the 1988-1999 to 1999-2010 eras
Here's the map of consistent weather stations in the United States - with a few scattered in southern Canada - that reported at least one temperature every season from 1988 to 2010. If you go on comment boards around the Internet, there are people who post with confidence that global warming has stopped and the world has not gotten warmer since 1998.
The data shown here does not agree with that statement. I use the method of comparing two eras that start and end in strong La Niña years with a strong El Niño in between. I could switch and use strong El Niño years and the endpoints with a strong La Niña in between, but that would not bring us as close to present day as this method does.
What can be said is that the warming trend slowed down using this measurement system. Here are the numbers from the United States stations compared to the data from the rest of the world.
Total stations:
U.S.A.: 3094
Rest of world 3363
Warming stations vs. cooling stations
U.S.A.: 71.5% warming, 28.5% cooling
Rest of world: 79.0% warming, 21.0% cooling
Average temperature change:
U.S.A.: 0.19° C
Rest of world: 0.29° C
This is still a warming trend, but not as fast as we saw comparing 1975-1988 to 1988-1999. If this continues, this would mean about a degree rise Celsius in the U.S.and a degree and half everyplace else. This is under the two degree per century threshold which climate scientists consider catastrophic.
Notice that the rest of the world has finally caught up and surpassed the United States in total consistent weather stations. A regularly reporting weather station is a useful thing, but not a vital part of the infrastructure. It's something of a luxury and back in 1955 the United States far outstripped the rest of the planet in being able to afford such luxuries, the aftereffects of World War II ten years earlier still being felt by the countries where the battles were fought. As so many statistical measurements tell us, we are no longer number one in the world in quality of life.
Another number that jumps out at me is that no matter how many stations report in the United States and how many stations report every place else, the ratio of the temperature change stays relatively constant, the rest of the world warming a little more than 50% faster than the U.S. This could just be coincidence, but it would be interesting to find out why. If there is a cause other than random chance, it might mean the United States is doing something other countries could emulate to bring their warming trends down.
After several weeks work, I am convinced climate change is real. The simplest way to explain it is the planet is getting warmer in general, but certainly not at the same rate everywhere. Just looking at the temperatures does not give any answer to the question of whether mankind's actions have any effect on the increase we are seeing; that takes modeling and modeling such a complex system has to be inexact, even on the incredibly powerful computers we have in the 21st Century. But if there is a man-made cause for temperatures rising slower in the United States than everywhere else, it would be in our best interest financially and environmentally to export that cause to the rest of the world.
The data shown here does not agree with that statement. I use the method of comparing two eras that start and end in strong La Niña years with a strong El Niño in between. I could switch and use strong El Niño years and the endpoints with a strong La Niña in between, but that would not bring us as close to present day as this method does.
What can be said is that the warming trend slowed down using this measurement system. Here are the numbers from the United States stations compared to the data from the rest of the world.
Total stations:
U.S.A.: 3094
Rest of world 3363
Warming stations vs. cooling stations
U.S.A.: 71.5% warming, 28.5% cooling
Rest of world: 79.0% warming, 21.0% cooling
Average temperature change:
U.S.A.: 0.19° C
Rest of world: 0.29° C
This is still a warming trend, but not as fast as we saw comparing 1975-1988 to 1988-1999. If this continues, this would mean about a degree rise Celsius in the U.S.and a degree and half everyplace else. This is under the two degree per century threshold which climate scientists consider catastrophic.
Notice that the rest of the world has finally caught up and surpassed the United States in total consistent weather stations. A regularly reporting weather station is a useful thing, but not a vital part of the infrastructure. It's something of a luxury and back in 1955 the United States far outstripped the rest of the planet in being able to afford such luxuries, the aftereffects of World War II ten years earlier still being felt by the countries where the battles were fought. As so many statistical measurements tell us, we are no longer number one in the world in quality of life.
Another number that jumps out at me is that no matter how many stations report in the United States and how many stations report every place else, the ratio of the temperature change stays relatively constant, the rest of the world warming a little more than 50% faster than the U.S. This could just be coincidence, but it would be interesting to find out why. If there is a cause other than random chance, it might mean the United States is doing something other countries could emulate to bring their warming trends down.
After several weeks work, I am convinced climate change is real. The simplest way to explain it is the planet is getting warmer in general, but certainly not at the same rate everywhere. Just looking at the temperatures does not give any answer to the question of whether mankind's actions have any effect on the increase we are seeing; that takes modeling and modeling such a complex system has to be inexact, even on the incredibly powerful computers we have in the 21st Century. But if there is a man-made cause for temperatures rising slower in the United States than everywhere else, it would be in our best interest financially and environmentally to export that cause to the rest of the world.
Tuesday, April 23, 2013
U.S. vs. the Rest of the World:
Climate change as measured from the 1975-1988 to 1988-1999 eras
Yesterday, we looked at the eras 1955-1975 compared to 1975-1988. Moving forward, now we look at 1975-1988 compared to 1988-1999.
In the United States, the warming trend increased quite a bit compared to the earlier era differences. 80% of weather stations that reported every season showed a warming trend and the average increase was 0.3° C compared to 0.09° C, and this increase takes place over a shorter period of time.
Here are the numbers of the U.S. vs, the rest of the world.
Total stations:
U.S.A.: 3534
Rest of world 3046
Warming stations vs. cooling stations
U.S.A.: 80.4% warming, 19.6% cooling
Rest of world: 86.2% warming, 13.8% cooling
Average temperature change:
U.S.A.: 0.30° C
Rest of world: 0.47° C
These changes are much more extreme than similar comparisons between the earlier eras reported yesterday. Climate scientists consider about two degrees Celsius in a century to be catastrophic warming. Since this is a twenty five year time span, the United States rate stretched to over a century would not be alarming, but the rest of the world would be.
Tomorrow, the 1988-1999 era compared to 1999-2010 era, a time when some global warming skeptics say the earth began cooling.
Monday, April 22, 2013
U.S. vs. the Rest of the World:
Climate change as measured from the 1955-1975 to 1975-1988 eras
Unless I get a brilliant idea, I'm going to post three more climate data entries and then I will go back to other topics in math. Here are my reasons.
1. I am now convinced that anyone who talks about "global cooling" is a liar.
2. The data I work with can only verify if the general climate is warming, cooling or staying the same. I can't make any statement about human cause and distrust predictions about the future, even when they were done with mathematical modeling. Since the climate shows itself to be warming over so many regions, that is the only question of interest I can answer.
3. My hope was to become part of the conversation and to get people on both sides to agree on ways to discuss the data that does not involve cherry picking. If I magically gained some kind of influence, I think I could talk to climate scientists. Warming denialists are a completely different kettle of fish, and a right putrid one at that.
Here is the first data from my last idea, unless some brilliant clue strikes me. By using the consistent weather stations around the world that report every season between two La Niña peaks that have El Niño peaks between them, I can look at the worldwide data trends. If I start in 1955, the United States has a huge advantage over the rest of the world. Having a consistently reporting weather station is a trifle in the developed world but a major cost in the undeveloped world. Even though World War II is ten years in the past and places like Canada, Australia and New Zealand come out as nearly unscathed as the U.S. does, the United States has more consistent weather stations than the rest of the world combined. Here is how the two groups compare.
Total stations:
U.S.A.: 3130
Rest of world 1938
Warming stations vs. cooling stations
U.S.A.: 60.9% warming, 39.1% cooling
Rest of world: 64.5% warming, 35.5% cooling
Average temperature change:
U.S.A.: 0.09° C
Rest of world: 0.14° C
This time period shows warming and the differences between the U.S. and the rest of the world are significant. Neither would be catastrophic is continued for a century, a rise of a less than a half degree Celsius.
Tomorrow, we move forward to 1975-1988 compared to 1988-1999.
Thursday, April 18, 2013
Taking a few days off.
I've tried to put up a post every day on the math blog this year, not with perfect success. I have some personal business to attend to over the next few days, so I'm taking today and Friday off for sure. I will try to put something up this weekend, Saturday at the earliest, setting myself a deadline of Sunday.
Wednesday, April 17, 2013
Distribution of temperature changes, U.S. vs. Worldwide
I published this map of weather station in the U.S. that reported consistently from Winter of 1955 to Fall of 2010. The color of the dots represent the difference in the era averages of 1999 to 2010 minus the average from 1955 to 1975. The darkest dots show severe warming, the hollow red dots show warming of less than a degree Celsius and the blue dots show stations that cooled.
Red dots outnumber blue dots, but by how much?
Here are the numbers put on a bar chart. The bar chart looks to be somewhat normally distributed and the average is in the moderate warming range. The severe warming stations slightly outnumber the stations that are cooling.
The worldwide differences still show the average to be in the moderate range, but there are more stations showing severe warming and less showing cooling trends.
In a set of numbers like this, two questions should be asked.
1. Are we measuring something important or not?
2. Could the differences we see just be random chance or is there an underlying reason?
If the measurement is important and it's not just random variation, it could mean that something the United States has been doing for the last half century or so might help slow down the warming trend seen in so many places.
Tuesday, April 16, 2013
Consistent weather station map:
Northern Hemisphere 1955-2010
I apologize for not posting yesterday. This blog takes thought sometimes and the news got in the way of concentration.
The dots on this map represent weather stations that reported at least one temperature every season from the Winter of 1955 to the Fall of 2010 in the Northern Hemisphere. The right side of the horizontal axis is the Prime Meridian, the left side is 180 degrees East and 180 degrees West. It's a little hard to make out shapes except for that clump in the low middle, which is obviously the United States of America.
The start of the era is very significant in terms of how many weather stations around the world will be reporting. In 1955, the list of true First World countries is USA. Europe is still digging out from the rubble of the war that ended ten years before and likewise Japan and much of Asia.
Let's take a closer look at the data from the United States.
There are four different kinds of marks on this map.
1. Dark with red outline. Strong warming stations. Here the average temperature increase from the 1955-1975 era to the 1999-2010 era is more than 1 degree Celsius. That's about 30% of all the stations.
2. Empty with red outline. Warming, but less than a degree Celsius. 60% of all stations.
3. Empty with blue outline. Cooling by less a degree Celsius. 10% of all stations
4. Dark blue with black outline. There are only a few on this map and they are hard to see at this magnification. A total of 5 out of 2,860, they don't even round to a percent.
Simply put, the large majority of the stations measured are showing warming, but by no means all. The average increase is 0.7 degrees Celsius with a standard deviation of 0.57 degrees. That's a lot of deviation, which means a lot of uncertainty. Climate denialists would play up that uncertainly and try to ignore the warming. As an observer outside the bar fight that is climate science right now, I wish someone would look at the cooling stations and figure out why. Maybe is just randomness, but maybe many of these stations have something in common that we might exploit to start a man-made global cooling effect that offsets what we do with out burning of fossil fuels.
I despair of the Culture of Constant Conflict that so much of human discourse has become. Climate science is one of those areas of discourse and the split it between the center left and the far right. (Conservatives including Margaret Thatcher and Arnold Schwarzenegger are with the current scientific consensus, as was Newt Gingrich before someone offered him more cash.)I'm just a beginner in the field, but I do have some ideas for research I'd like to see explored and this is one of them.
A new idea: What happens if we make the early era 1975-1988? All consistent stations in this set will still be consistent, but several others should join the ranks and the differences in the two averages cannot be the same.
More on this later this week.
The dots on this map represent weather stations that reported at least one temperature every season from the Winter of 1955 to the Fall of 2010 in the Northern Hemisphere. The right side of the horizontal axis is the Prime Meridian, the left side is 180 degrees East and 180 degrees West. It's a little hard to make out shapes except for that clump in the low middle, which is obviously the United States of America.
The start of the era is very significant in terms of how many weather stations around the world will be reporting. In 1955, the list of true First World countries is USA. Europe is still digging out from the rubble of the war that ended ten years before and likewise Japan and much of Asia.
Let's take a closer look at the data from the United States.
There are four different kinds of marks on this map.
1. Dark with red outline. Strong warming stations. Here the average temperature increase from the 1955-1975 era to the 1999-2010 era is more than 1 degree Celsius. That's about 30% of all the stations.
2. Empty with red outline. Warming, but less than a degree Celsius. 60% of all stations.
3. Empty with blue outline. Cooling by less a degree Celsius. 10% of all stations
4. Dark blue with black outline. There are only a few on this map and they are hard to see at this magnification. A total of 5 out of 2,860, they don't even round to a percent.
Simply put, the large majority of the stations measured are showing warming, but by no means all. The average increase is 0.7 degrees Celsius with a standard deviation of 0.57 degrees. That's a lot of deviation, which means a lot of uncertainty. Climate denialists would play up that uncertainly and try to ignore the warming. As an observer outside the bar fight that is climate science right now, I wish someone would look at the cooling stations and figure out why. Maybe is just randomness, but maybe many of these stations have something in common that we might exploit to start a man-made global cooling effect that offsets what we do with out burning of fossil fuels.
I despair of the Culture of Constant Conflict that so much of human discourse has become. Climate science is one of those areas of discourse and the split it between the center left and the far right. (Conservatives including Margaret Thatcher and Arnold Schwarzenegger are with the current scientific consensus, as was Newt Gingrich before someone offered him more cash.)I'm just a beginner in the field, but I do have some ideas for research I'd like to see explored and this is one of them.
A new idea: What happens if we make the early era 1975-1988? All consistent stations in this set will still be consistent, but several others should join the ranks and the differences in the two averages cannot be the same.
More on this later this week.
Sunday, April 14, 2013
New data method:
Consistent weather station map
I had a new idea for how to look at the big data set made available by Berkeley Earth Surface Temperature. While I now understand the effects of La Niña and El Niño are not worldwide, they do make a difference over a huge amount of the earth's surface so I will continue to consider eras that start and end with a strong La Niña years (or conversely with a strong El Niño years) to be time periods that are worth comparing.
I took the data set and wrote a C program that is interested only in the weather stations worldwide that reported a temperature for every season from 1955 to 2010, both of which are strong La Niña years. The earliest La Niña to La Niña era is 1955 to 1975, while the most recent spans from 1999 to 2010. Complete data for 2011 and 2012 is dribbling in, but neither is measured as strong La Niña or strong El Niño, so these are the time periods I use to avoid cherry picking the data, which will often mean the data is not completely up to date.
Tomorrow, I will start showing the results for these consistent weather stations, looking at the difference of the averages of the early and late eras. Splitting the data into the two hemispheres, the northern hemisphere is much better covered than the south, completely unsurprising given the differences in both land mass and population. There are many ways to look at a data set this big and as the week progresses we will go from the simplest ideas to the more complex.
Saturday, April 13, 2013
Climate Data:
Siberia 1955-2010
The split between Siberia and the rest of Russia is usually defined as the Ural Mountains. Since I wanted to make it a rectangle is longitude and latitude, I chose 60 degree East for the leftmost cutoff point.
We think of Siberia as "frozen wasteland" and "place of exile", but I have read several Russian writer who say it has a meaning similar to the West in United States' cultural memory, a big, mostly empty place of challenge and opportunity.
Whichever it is, it certainly is not wanting for weather stations to measure its progress. Very good coverage from east to west and north to south.
The temperatures in Winter vary drastically. While the warmest Winters have spiked pretty close to each other all through the 1955-2010 era, the coldest Winters are getting generally warmer and the median keeps sneaking upward era by era.
Spring does not show as much fluctuation and the sequences show more steady increase.
Summer fluctuates even less and every measurement method shows consistent increase, but the total increase of the median from the 1955-1975 era to the 1999-2010 era is less than a degree Celsius, the mildest increase of all four seasons.
Like Winter, Fall temperatures jump around a lot. The warmest Fall are only slightly warmer, but the median has climbed over a degree and a half.
Confidence level of warming from time interval to time interval: 99.99%
Confidence level of the trend showing increasing warming: 65.8%
Average seasonal change in the medians of 1955-1975 to 1999-2010: 1.555° C.
1.555° C in 56 years is the number that matters. This is way too fast. More than that, the Siberian permafrost, like the Winter ice in the Arctic Circle, can become a really scary feedback loop. There's a lot of permafrost in Siberia, and most importantly there is a contiguous permafrost about the size of France and Germany combined. When permafrost melts, it changes the albedo, absorbing heat instead of reflecting it. If that wasn't bad enough, there is a huge amount of CO2 and methane trapped in the permafrost that will be released when it melts, things will only get worse.
I've only been looking at climate data for about ten weeks now, but I am pretty close to convinced it's real and it does not have to speed up for it to be a serious problem for humans, if not in my lifetime then in the lifetime of kids growing up today. Tomorrow, I will introduce another way to look at the data. I have not done the programming yet, so I don't know if the data will alarming or not, but it is an attempt to look at patterns worldwide instead of getting useful data region by region.
Friday, April 12, 2013
Climate Data:
Sonoran Desert 1955-2010
The Sonoran Desert spans much of Northwestern Mexico and parts of California and Arizona. The best known cities north of the border are Phoeniz and Tuscon.
The coverage by consistent weather stations is much stronger in the United States than it is in Mexico. Those grid marks in the lower right hand corner are from the tip of Baja California.
While the record high temperature for Winter average is now nearly 20 years old, the other ways of measuring - the median in the red dotted line and the coldest winter, marked by the lower black line - are showing marked increase.
The Spring data is increasing using every measurement system shown here.
And the Summer is as well.
More than just steady increase, every season has shown a median increase from the 1955-1975 era to the 1999-2010 era of more that a degree Celsius, which is the simplest number that marks the Big Damn Deal cut-off point.
Confidence level of warming from time interval to time interval: 99.999%
Confidence level of the trend showing increasing cooling or warming: 50%
Average seasonal change in the medians of 1955-1975 to 1999-2010: 1.38° C.
The data says it's getting warmer but the trend is not currently on the upswing. This is some good news but not a lot, as an increase of 1.38° C in 56 years is already fast enough to be considered catastrophic.
Thursday, April 11, 2013
Climate Data: Atacama Desert 1955-2010
The Atacama Desert in South America is said to be the driest place on earth. Much of it high in the mountains, there are regions so desolate they look like they could be on the moon.
It is possible to have weather stations that are not manned, but it is rare. The inhospitable locations and climate of the Atacama means there is only one weather station that has reported consistently from 1955 to 2010.
Because this station is in the Southern Hemisphere, the beginning of the year is Summer. There is no strong trend, with highs and lows bouncing around randomly. The most recent era's median is slightly lower than the 1955-1975 median.
Change in 1955-1975 median to 1999-2010 median: -0.04° C.
The Fall temperatures from this station show a steep cooling trend this century after slight warming last century.
Change in 1955-1975 median to 1999-2010 median: -0.38° C.
Winter is like Fall, but with a much steeper recent drop.
Change in 1955-1975 median to 1999-2010 median: -1.47° C.
The Spring data also shows the recent drop.
Change in 1955-1975 median to 1999-2010 median: -1.31° C.
Confidence level of cooling from time interval to time interval: 63%
Confidence level of the trend showing increasing cooling: 88.9%
Average seasonal change in the medians of 1955-1975 to 1999-2010: -0.80° C.
As stated earlier, this is really just the data from a single station, not from an entire region. That said, this station shows a cooling trend over our 56 year only matched by a part of eastern Antarctica in our earlier worldwide survey.
This gives me an idea for way to search the data, looking for completely consistent stations worldwide over the 1955 to 2010 time period and checking the difference in the first era defined by the Consistent Oceanic Nino Intervals (1955 to 1975) and the most recent era (1999 to 2010).
But for now, I will continue to look at certain deserts. Tomorrow, the Sonoran Desert, which straddles the border of the western United States and Mexico.
Wednesday, April 10, 2013
Climate Data: Mongolia 1955-2010
During my four weeks of climate data, the regions I selected followed a set pattern. Right now, I am hopping around the map with no consistent rhyme or reason. I chose the Sahara and Australia because they are both dominated by desert. I chose China because I wanted to see what was happening in a very populated area. I choose Mongolia today because it fits nicely in a longitude/latitude rectangle, it was part of the China map yesterday and it contains a large part of the Gobi Desert, continuing with the theme of deserts started earlier.
Mongolia is much more sparsely populated than China and not as well covered by weather stations. Even so, there were 23,627 seasonal reports between 1955 to 2010, so it is nothing like the paucity of information we had in the polar regions.
Average Winter temperatures are all over the place, from less than 2° C to nearly 10° C. These temperatures are much colder than China and much more variable. The general trend is upward, though there was a drop in the low temperature and median at the beginning of this century compared to the end of the last.
Change in 1955-1975 median to 1999-2010 median: 1.6925° C.
Spring shows steadily climbing in all our measurement standards, though the amount of increase is slowing down.
Change in 1955-1975 median to 1999-2010 median: 1.877° C.
Summer shows increasing temperatures and the rate of warming is going up.
Change in 1955-1975 median to 1999-2010 median: 1.46° C.
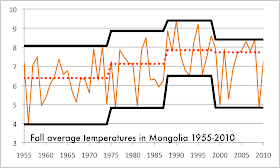
Fall shows a temperature dip between 1988-1999 to 1999-2010, but these temperatures are still over a degree higher than what was seen in the 1955-197 era.
Change in 1955-1975 median to 1999-2010 median: 1.35° C.
===
Confidence level of warming from time interval to time interval: 99.98%
Confidence level of the trend showing increasing warming: 65.8%
Average seasonal change in the medians of 1955-1975 to 1999-2010: 1.59° C.
We are very confident that this is a warming trend and not just random variation. We are not confident at all that the warming is speeding up, but at 1.59° C in 56 years, we don't have to be. This number is definitely in the "hair on fire" range. Of the regions I've looked at, this is the worst increase of anyplace outside the polar regions.
This gets me interested in deserts in general. Tomorrow: the Atacama desert in South America.
Mongolia is much more sparsely populated than China and not as well covered by weather stations. Even so, there were 23,627 seasonal reports between 1955 to 2010, so it is nothing like the paucity of information we had in the polar regions.
Average Winter temperatures are all over the place, from less than 2° C to nearly 10° C. These temperatures are much colder than China and much more variable. The general trend is upward, though there was a drop in the low temperature and median at the beginning of this century compared to the end of the last.
Change in 1955-1975 median to 1999-2010 median: 1.6925° C.
Spring shows steadily climbing in all our measurement standards, though the amount of increase is slowing down.
Change in 1955-1975 median to 1999-2010 median: 1.877° C.
Summer shows increasing temperatures and the rate of warming is going up.
Change in 1955-1975 median to 1999-2010 median: 1.46° C.
Fall shows a temperature dip between 1988-1999 to 1999-2010, but these temperatures are still over a degree higher than what was seen in the 1955-197 era.
Change in 1955-1975 median to 1999-2010 median: 1.35° C.
===
Confidence level of warming from time interval to time interval: 99.98%
Confidence level of the trend showing increasing warming: 65.8%
Average seasonal change in the medians of 1955-1975 to 1999-2010: 1.59° C.
We are very confident that this is a warming trend and not just random variation. We are not confident at all that the warming is speeding up, but at 1.59° C in 56 years, we don't have to be. This number is definitely in the "hair on fire" range. Of the regions I've looked at, this is the worst increase of anyplace outside the polar regions.
This gets me interested in deserts in general. Tomorrow: the Atacama desert in South America.